Almost Locked Pair

This is a nice little pattern that is simple enough to be included in the Tough strategies section. Promoted by Gordon Fick on the New Sudoku Forum, his thread is here. The same pattern is discovered using more sophisticated methods down the list but I think is worth spotting earlier on. It is a "bent-set" and should be another Wing type in my opinion.

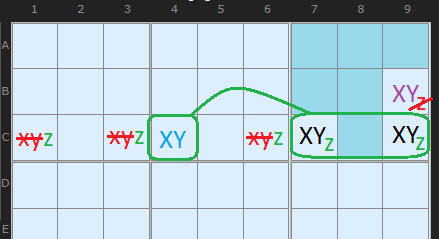
Two numbers X and Y are both in a box and aligned on a row or column. In the first diagram they are orientated on row C. The pattern has two orientations. In the first the box contains only one other place where X or Y can go, namely B9 which means the Grouped Cell C7/C9 must contain at least one of X or Y. 'Z' are extra candidates that appear those cells.

Looking along the row there is a bi-value cell C4 permitting only X or Y. Since X or Y must appear in that cell at most only one of X or Y can appear in the Grouped Cell C7/C9.

As Gordon writes, the idea that C7/C9 must contain at least and at most one of X or Y makes for a Virtual Locked Pair with C4. In the box any other candidate in B9 can be removed and all X an Y in row C not in the Virtual Locked Pair can be removed.

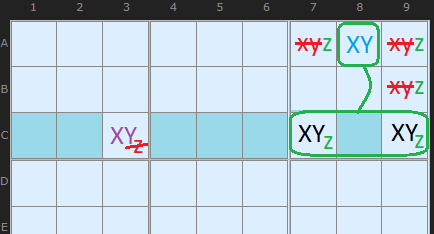
The second orientation swaps the bi-value X/Y cell with the bi-location X/Y. Now the row contains the three cells meaning C7/C9 has at least one of X and Y (making it bi-location since it is a single cell C3 plus a Grouped Cell C7/C9).

Because the bi-value cell A8 must contain one of X or Y the Grouped Cell can have at most one of X or Y forming the Virtual Locked Pair.

This all of X and Y can be removed from the rest of box 3 and any extra candidates on C3 can be eliminated.

to do